| University | National University of Singapore (NUS) |
| Subject | MA1301 Introductory Math |
- Do NOT upload this assignment problem set to any website.
- The assignment carries a total number of 100 marks. The marks for each question or part are as indicated.
(1) (a) [5 marks] The first term of an arithmetic sequence is 7, its last term in 70 and its sum is 385. Find the number of terms in the sequence and the common difference
(b) A geometric series is given as follows:
![]()
(i) [5 marks] Find the set of values of x such that the sum to infinity of the series exists (in other words, the series converges).
(ii) [5 marks] Find the value of x for which the sum to infinity
of the series S∞ is 43.
(c) [5 marks] An arithmetic sequence {an} ∞ n=1 of positive terms is such that twice of the sum of the first nine terms is equal to the sum of the next nine terms. Furthermore, a1, 20, a16 forms a geometric sequence. Find the first term and the common difference of the arithmetic sequence.
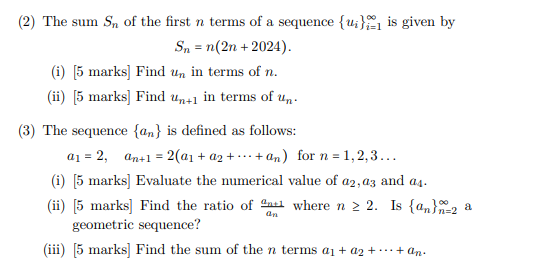
(2) The sum Sn of the first n terms of a sequence {ui}
∞ i=1 is given by Sn = n(2n + 2024).
(i) [5 marks] Find un in terms of n.
(ii) [5 marks] Find un+1 in terms of un.
(3) The sequence {an} is defined as follows:
a1 = 2, an+1 = 2(a1 + a2 + ⋅ ⋅ ⋅ + an) for n = 1, 2, 3 . . .
(i) [5 marks] Evaluate the numerical value of a2, a3 and a4.
(ii) [5 marks] Find the ratio of an+1
an where n ≥ 2. Is {an}∞n=2ageometric sequence?
(iii) [5 marks] Find the sum of the n terms a1 + a2 + ⋅ ⋅ ⋅ + an.
Hire a Professional Essay & Assignment Writer for completing your Academic Assessments
Native Singapore Writers Team
- 100% Plagiarism-Free Essay
- Highest Satisfaction Rate
- Free Revision
- On-Time Delivery
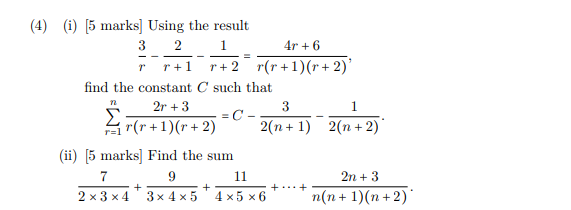
(5) (a) [5 marks] Let n be a positive integer with n > 1. Suppose that the coefficient of x 3 in the expansion of (1 + x) 2n and the coefficient of x 2 in the expansion of (1 + 10x) n are equal. Find the value of n.
(b) [5 marks] Let k > 0 be a constant. Suppose the coefficient of x2 in the expansion of

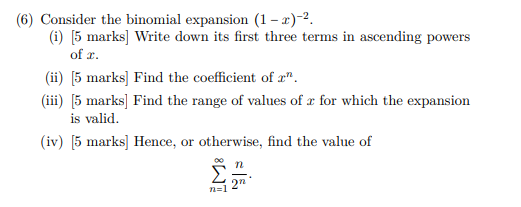
(6) Consider the binomial expansion (1 − x)−2
(i) [5 marks] Write down its first three terms in ascending powersof x.
(ii) [5 marks] Find the coefficient of xn.
(iii) [5 marks] Find the range of values of x for which the expansion is valid.
(iv) [5 marks] Hence, or otherwise, find the value of
∞∑n=1n2
(7) (i) [5 marks] Expand (1−x1+x)n in ascending powers of x up to and including the term in x2.
(ii) [5 marks] State the set of values of x for which the series expansion is valid.
(iii) [5 marks] Hence find an approximation to the fourth root of 19 21 , in the form p q, where p and q are positive integers with no common factors.

Stuck with a lot of homework assignments and feeling stressed ? Take professional academic assistance & Get 100% Plagiarism free papers
Facing challenges with your MA1301 Introductory Math Assignment? Let our assignment writing services assist you. Singaporean students can rely on our homework writing helper online for students, providing original content and ensuring your work is done on time. Simply ask us to do my assignment for me, and we’ll handle the rest!
Looking for Plagiarism free Answers for your college/ university Assignments.
- HRM331: Talent Management – Strategic Shift from the War for Talent to the Wealth of Talent
- Marginalised Populations – The Structural and Cultural Exclusion of People Experiencing Homelessness in Singapore
- CVEN3501 Assignment 2: Groundwater Modelling of Drawdown from a Pumping Bore
- CSCI312 Assignment 2: Conceptual Modelling and Implementation of a Data Warehouse and Hive Queries
- CH2123 Assignnment : Fugacity, VLE Modeling & Henry’s Law Applications
- BAFI1045 Assignment -Constructing and Evaluating Passive and Active Portfolios Based on the Straits Times Index (STI)
- PSB501EN Assignment 1: Engineering Systems Integration: A Multi-Technique Approach to Mechanical Analysis
- FIN2210E/FIN2212E Group Assignment: Financial Risk Management Analysis of Bursa Malaysia Companies
- FLM101 Assignment: A Cinematic Dissection: Stylistic Elements and Their Thematic Significance
- Assignment: Transforming Talent in the AI Era: From War to Wealth through Ecosystem Innovation

